This post was originally posted in 2012. It is still very popular, so I wanted to share it here in case you get some value from it.
Here’s a laser power density calculator, if that’s what you wanted.
Laser engineers and technicians are often required to calculate a laser’s power density to determine whether a beam would damage an optic or sensor or for other various applications. By definition, power density is power per unit area which is usually expressed in terms of W/cm2.
In this Blog we’ll explain the details of how power density depends on the beam profile of your laser and how to calculate it using our power density calculator.
The Calculation of power density is straightforward. By simply calculating the area of a beam using the radius in cm and dividing the beam’s power by that area the power density in units of W/cm2 is easily obtained.
However, since beam size is usually given with the beam diameter in terms of millimeters to calculate power density in W/cm2 a user has to convert the diameter to cm, divide the diameter by two to find the radius, use πr2 to find the area in cm2, and finally divide the laser power by the area to obtain power density. This calculation can be a bit tedious and time consuming especially for technicians and field engineers who want to make as quick and easy a calculation as possible.
httpv://youtu.be/U2oliO-Cz8M
With the following formula you can directly find power density of a laser beam using the diameter of the beam in millimeters:
Here is how this equation is derived: We can write an expression for the power density of a1 mmdiameter beam, which is simply:
Dividing the expression of power density of a 1 mmbeam — Power / π(0.5mm)2 — by an expression of power density as a function of diameter — Power / π(0.5d)2 — we find that the ratio is d2. Thus, power density as a function of diameter can be expressed as the power density of a 1 mm beam divided by d2:
This formula assumes the beam is a flat top beam where the beam power density is uniform. For a Gaussian beam with a given beam waist in mm, multiply this formula by two to obtain the formula given above (The multiplication factor is due to the fact that for a Gaussian beam, the peak power in the center is twice the average power density of the beam. The value is actually closer to 255, not 250, but this difference is trivial, introducing only ~2% error. We use 250 simply because it’s easier to remember and perform mental calculations than 255! )
What other laser parameters are annoying to calculate? Let me know in the comments.
Source: technical note from Newport corporations Website
You might also like to read: How to measure the peak power of a pulsed laser?
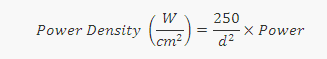
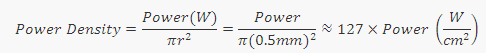
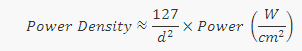










Leave a Reply
Your email address will not be published. Required fields are marked *